![]() D.4 Derivation
of the PDE
D.4 Derivation
of the PDE
We
start with a more general argument. Let
f(S,t) denote the value of any derivative security (e.g., a call
option) at time t when the stock price
is S. Note that, by assumption,
the derivative price does not depend on the path of S, but only on the current value of S.
By
Ito's lemma,

and
recall that for the stock price
![]()
Now,
consider the riskless hedge portfolio in Chapter 2, topic 2.4.
-k
derivative security
1
share of stock.
The
number of derivative securities, k, is
![]() .
.
The
value of this portfolio, V, is given
by
![]()
and
the change in the value is
![]()
Substituting
the df equation and the dS equation into this portfolio and rearranging, we get
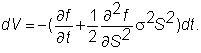
The
important fact about this equation is that dV
is independent of the stochastic term z.
This means that all the risk of the derivative can be hedged by holding
![]()
units
of the derivative.
Since
dV is instantaneously deterministic, the portfolio return must equal
the instantaneous risk-free return. In
other words,
![]()
Substituting
for dV and V, we get
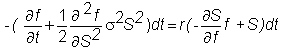
or
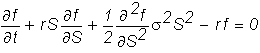
This
is called the Black-Scholes (partial) differential equation.
The solution to this equation, subject to the appropriate boundary
condition for f, determines the value
of the derivative security. For a
European call option, the boundary condition is
f(S,T)
= max{0,S-X}.
The
solution of the differential equation for the call option is given by the Black-Scholes
formula:
![]()
where
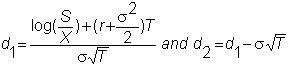
We
can also apply Ito’s Lemma to derive the implied distribution of stock prices
from a geometric Brownian motion model of stock prices.
The next topic Distribution of Stock
Prices provides a technical overview of this issue.
(C) Copyright 1999, OS
Financial Trading System